An elegant proof of the Hardy-Weinberg ratio can be given in terms of gametes.

Diploid organisms produce haploid gametes. We could imagine that the haploid gametes are all released into the sea, where they combine at random to form the next generation. This is called random union of gametes.

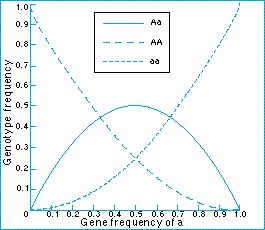
In the 'gamete pool' A gametes will have frequency p and a gametes frequency q . Because they are combining at random, an a gamete will meet an A gamete with chance p and an a gamete with chance q. From the a gametes, Aa zygotes will therefore be produced with frequency pq and aa gametes with frequency q .

A similar argument applies for the A gametes (which have frequency p ): they combine with a gametes with chance q, to produce Aa zygotes (frequency pq ) and A gametes with chance p to form AA zygotes (frequency p² ). If we now add up the frequencies of the genotypes from the two types of gamete, the Hardy-Weinberg genotype frequencies emerge.

Figure: Hardy-Weinberg proportions of genotypes AA, Aa and aa in relation to the frequency of the gene a.